Homework 4 Solutions
Solution Files
You can find the solutions in hw04.py.
Required Questions
Getting Started Videos
These videos may provide some helpful direction for tackling the coding problems on this assignment.
To see these videos, you should be logged into your berkeley.edu email.
Generators
Q1: Infinite Hailstone
Write a generator function that yields the elements of the hailstone sequence starting at number n.
After reaching the end of the hailstone sequence, the generator should yield the value 1 indefinitely.
Here's a quick reminder of how the hailstone sequence is defined:
- Pick a positive integer
nas the start. - If
nis even, divide it by 2. - If
nis odd, multiply it by 3 and add 1. - Continue this process until
nis 1.
Try to write this generator function recursively. If you're stuck, you can first try writing it iteratively and then seeing how you can turn that implementation into a recursive one.
Hint: Since
hailstonereturns a generator, you canyield froma call tohailstone!
def hailstone(n):
"""Q1: Yields the elements of the hailstone sequence starting at n.
At the end of the sequence, yield 1 infinitely.
>>> hail_gen = hailstone(10)
>>> [next(hail_gen) for _ in range(10)]
[10, 5, 16, 8, 4, 2, 1, 1, 1, 1]
>>> next(hail_gen)
1
"""
yield n
if n == 1:
yield from hailstone(n)
elif n % 2 == 0:
yield from hailstone(n // 2)
else:
yield from hailstone(n * 3 + 1)Use Ok to test your code:
python3 ok -q hailstoneQ2: Merge
Write a generator function merge that takes in two infinite generators a and b that are in increasing order without duplicates and returns a generator that has all the elements of both generators, in increasing order, without duplicates.
def merge(a, b):
"""Q2:
>>> def sequence(start, step):
... while True:
... yield start
... start += step
>>> a = sequence(2, 3) # 2, 5, 8, 11, 14, ...
>>> b = sequence(3, 2) # 3, 5, 7, 9, 11, 13, 15, ...
>>> result = merge(a, b) # 2, 3, 5, 7, 8, 9, 11, 13, 14, 15
>>> [next(result) for _ in range(10)]
[2, 3, 5, 7, 8, 9, 11, 13, 14, 15]
"""
first_a, first_b = next(a), next(b)
while True:
if first_a == first_b:
yield first_a
first_a, first_b = next(a), next(b)
elif first_a < first_b:
yield first_a
first_a = next(a)
else:
yield first_b
first_b = next(b)Use Ok to test your code:
python3 ok -q mergeQ3: Generate Permutations
Given a sequence of unique elements, a permutation of the sequence is a list
containing the elements of the sequence in some arbitrary order. For example,
[2, 1, 3], [1, 3, 2], and [3, 2, 1] are some of the permutations of the
sequence [1, 2, 3].
Implement perms, a generator function that takes in a sequence seq
and returns a generator that yields all permutations of seq. For this question,
assume that seq will not be empty.
Permutations may be yielded in any order. Note that the doctests test whether
you are yielding all possible permutations, but not in any particular order.
The built-in sorted function takes in an iterable object and returns a list
containing the elements of the iterable in non-decreasing order.
Hint: If you had the permutations of all but one of the elements in
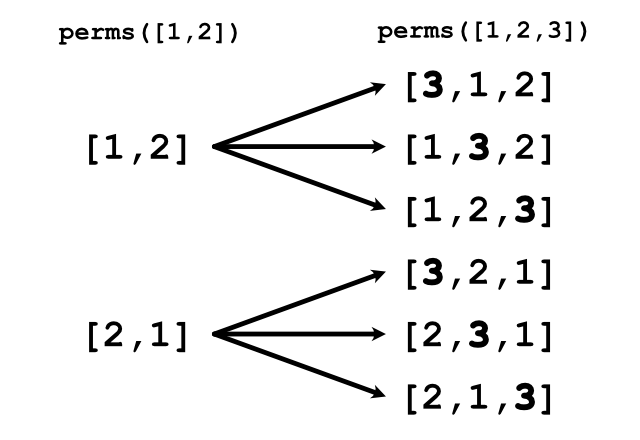
seq, how could you use that to generate the permutations of the fullseq? For example, you can useperms([1,2])to generate permutations forperms([1,2,3]):
Try drawing a similar diagram for
perms([1,2,3,4]).
Hint: Remember, it's possible to loop over generator objects because generators are iterators!
def perms(seq):
"""Q3: Generates all permutations of the given sequence. Each permutation is a
list of the elements in SEQ in a different order. The permutations may be
yielded in any order.
>>> p = perms([100])
>>> type(p)
<class 'generator'>
>>> next(p)
[100]
>>> try: # Prints "No more permutations!" if calling next would cause an error
... next(p)
... except StopIteration:
... print('No more permutations!')
No more permutations!
>>> sorted(perms([1, 2, 3])) # Returns a sorted list containing elements of the generator
[[1, 2, 3], [1, 3, 2], [2, 1, 3], [2, 3, 1], [3, 1, 2], [3, 2, 1]]
>>> sorted(perms((10, 20, 30)))
[[10, 20, 30], [10, 30, 20], [20, 10, 30], [20, 30, 10], [30, 10, 20], [30, 20, 10]]
>>> sorted(perms("ab"))
[['a', 'b'], ['b', 'a']]
"""
if not seq:
yield []
else:
for p in perms(seq[1:]):
for i in range(len(seq)):
yield p[:i] + [seq[0]] + p[i:]Use Ok to test your code:
python3 ok -q permsQ4: Yield Paths
Define a generator function yield_paths which takes in a tree t, a value
value, and returns a generator object which yields each path from the root
of t to a node that has label value.
Each path should be represented as a list of the labels along that path in the tree. You may yield the paths in any order.
def yield_paths(t, value):
"""Q4: Yields all possible paths from the root of t to a node with the label
value as a list.
>>> t1 = tree(1, [tree(2, [tree(3), tree(4, [tree(6)]), tree(5)]), tree(5)])
>>> print_tree(t1)
1
2
3
4
6
5
5
>>> next(yield_paths(t1, 6))
[1, 2, 4, 6]
>>> path_to_5 = yield_paths(t1, 5)
>>> sorted(list(path_to_5))
[[1, 2, 5], [1, 5]]
>>> t2 = tree(0, [tree(2, [t1])])
>>> print_tree(t2)
0
2
1
2
3
4
6
5
5
>>> path_to_2 = yield_paths(t2, 2)
>>> sorted(list(path_to_2))
[[0, 2], [0, 2, 1, 2]]
"""
if label(t) == value:
yield [value] for b in branches(t):
for path in yield_paths(b, value): yield [label(t)] + pathHint: If you're having trouble getting started, think about how you'd approach this problem if it wasn't a generator function. What would your recursive calls be? With a generator function, what happens if you make a "recursive call" within its body?
Hint: Try coming up with a few simple cases of your own! How should this function work when
tis a leaf node?
Hint: Remember, it's possible to loop over generator objects because generators are iterators!
Note: Remember that this problem should yield paths -- do not return a list of paths!
Use Ok to test your code:
python3 ok -q yield_pathsIf our current label is equal to value, we've found a path from the root to a node
containing value containing only our current label, so we should yield that. From there,
we'll see if there are any paths starting from one of our branches that ends at a
node containing value. If we find these "partial paths" we can simply add our current
label to the beinning of a path to obtain a path starting from the root.
In order to do this, we'll create a generator for each of the branches which yields
these "partial paths". By calling yield_paths on each of the branches, we'll create
exactly this generator! Then, since a generator is also an iterable, we can iterate over
the paths in this generator and yield the result of concatenating it with our current label.
OOP
Q5: Minty
A mint is a place where coins are made. In this question, you'll implement a Minty class that can output a Coin with the correct year and worth.
- Each
Mintyinstance has ayearstamp. Theupdatemethod sets theyearstamp to thepresent_yearclass attribute of theMintyclass. - The
createmethod inMintyreturns an instance ofCoinstamped with theMintyinstance's year (which may be different fromMinty.present_yearif the stamp has not been updated.) - The
Coinconstructor should ensure that each coin has the correct year and denomination value (cents), specific to the type of coin (in our case, just 'Dime' or 'Nickel'). - A
Coin'sworthmethod returns itscentsvalue plus an additional cent for each year of age beyond 50 years. A coin's age can be determined by subtracting the coin's year from thepresent_yearclass attribute of theMintyclass.
Note: The getting started video for this problem covers a version that includes inheritance, but this problem does not involve inheritance.
class Minty:
"""A mint creates coins by stamping on years. The update method sets the mint's stamp to Minty.present_year.
>>> mint = Minty()
>>> mint.year
2021
>>> dime = mint.create('Dime')
>>> dime.year
2021
>>> Minty.present_year = 2101 # Time passes
>>> nickel = mint.create('Nickel')
>>> nickel.year # The mint has not updated its stamp yet
2021
>>> nickel.worth() # 5 cents + (80 - 50 years)
35
>>> mint.update() # The mint's year is updated to 2101
>>> Minty.present_year = 2176 # More time passes
>>> mint.create('Dime').worth() # 10 cents + (75 - 50 years)
35
>>> Minty().create('Dime').worth() # A new mint has the current year
10
>>> dime.worth() # 10 cents + (155 - 50 years)
115
"""
present_year = 2021
def __init__(self):
self.update()
def create(self, type):
return Coin(self.year, type)
def update(self):
self.year = Minty.present_year
class Coin:
cents = 50
def __init__(self, year, type):
self.year = year
if type == ('Dime'):
self.cents = 10
elif type == ('Nickel'):
self.cents = 5
def worth(self):
return self.cents + max(0, Minty.present_year - self.year - 50)Use Ok to test your code:
python3 ok -q MintyQ6: Vending Machine
In this question you'll create a vending machine that sells a single product and provides change when needed.
Create a class called VendingMachine that represents a vending
machine for some product. A VendingMachine object returns strings
describing its interactions. Make sure your output exactly matches the strings in the doctests including punctuation and spacing!
You may find Python's formatted string literals, or f-strings useful. A quick example:
>>> feeling = 'love' >>> course = '61A!' >>> f'I {feeling} {course}' 'I love 61A!'
Fill in the VendingMachine class, adding attributes and methods as appropriate, such that its behavior matches the following doctests:
class VendingMachine:
"""A vending machine that vends some product for some price.
>>> v = VendingMachine('candy', 10)
>>> v.vend()
'Nothing left to vend. Please restock.'
>>> v.add_funds(15)
'Nothing left to vend. Please restock. Here is your $15.'
>>> v.restock(2)
'Current candy stock: 2'
>>> v.vend()
'Please add $10 more funds.'
>>> v.add_funds(7)
'Current balance: $7'
>>> v.vend()
'Please add $3 more funds.'
>>> v.add_funds(5)
'Current balance: $12'
>>> v.vend()
'Here is your candy and $2 change.'
>>> v.add_funds(10)
'Current balance: $10'
>>> v.vend()
'Here is your candy.'
>>> v.add_funds(15)
'Nothing left to vend. Please restock. Here is your $15.'
>>> w = VendingMachine('soda', 2)
>>> w.restock(3)
'Current soda stock: 3'
>>> w.restock(3)
'Current soda stock: 6'
>>> w.add_funds(2)
'Current balance: $2'
>>> w.vend()
'Here is your soda.'
"""
def __init__(self, product, price):
self.product = product
self.price = price
self.stock = 0
self.balance = 0
def restock(self, n):
self.stock += n
return f'Current {self.product} stock: {self.stock}'
def add_funds(self, n):
if self.stock == 0:
return f'Nothing left to vend. Please restock. Here is your ${n}.'
# Alternatively, we could have:
# return self.vend() + f' Here is your ${n}.'
self.balance += n
return f'Current balance: ${self.balance}'
def vend(self):
if self.stock == 0:
return 'Nothing left to vend. Please restock.'
difference = self.price - self.balance
if difference > 0:
return f'Please add ${difference} more funds.'
message = f'Here is your {self.product}'
if difference != 0:
message += f' and ${-difference} change'
self.balance = 0
self.stock -= 1
return message + '.'Use Ok to test your code:
python3 ok -q VendingMachineReading through the doctests, it should be clear which functions we should add to ensure that the vending machine class behaves correctly.
__init__
- This can be difficult to fill out at first. Both
productandpriceseem pretty obvious to keep around, butstockandbalanceare quantities that are needed only after attempting other functions.
restock
- Even though
v.restock(2)takes only one argument in the doctest, remember thatselfis bound to the object therestockmethod is invoked on. Therefore, this function has two parameters. - While implementing this function, you will probably realize that you would
like to keep track of the stock somewhere. While it might be possible to set
the stock in this function as an instance attribute, it would lose whatever
the old stock was.
Therefore, the natural solution is to initialize stock in the constructor, and
then update it in
restock.
add_funds
- This behaves very similarly to
restock. See comments above. - Also yes, this is quite the expensive vending machine.
vend
The trickiest thing here is to make sure we handle all the cases. You may find it helpful when implementing a problem like this to keep track of all the errors we run into in the doctest.
- No stock
- Not enough balance
- Leftover balance after purchase (return change to customer)
- No leftover balance after purchase
We use some string concatenation at the end when handling case 3 and 4 to try and reduce the amount of code. This isn't necessary for correctness -- it's ok to have something like:
if difference != 0: return ... else: return ...Of course, that would require decrementing the balance and stock beforehand.
Check Your Score Locally
You can locally check your score on each question of this assignment by running
python3 ok --scoreThis does NOT submit the assignment! When you are satisfied with your score, submit the assignment to Gradescope to receive credit for it.
Submit Assignment
Submit this assignment by uploading any files you've edited to the appropriate Gradescope assignment. Lab 00 has detailed instructions.
Exam Practice
Homework assignments will also contain prior exam-level questions for you to take a look at. These questions have no submission component; feel free to attempt them if you'd like a challenge!
Generators
- Summer 2018 Final Q7a,b: Streams and Jennyrators
- Spring 2019 Final Q1: Iterators are inevitable
- Spring 2021 MT2 Q8: The Tree of L-I-F-E
- Summer 2016 Final Q8: Zhen-erators Produce Power
- Spring 2018 Final Q4a: Apply Yourself
Object-Oriented Programming
- Spring 2022 MT2 Q8: CS61A Presents The Game of Hoop.
- Fall 2020 MT2 Q3: Sparse Lists